Comparison of axial length, keratometry and refraction between DNEye Scanner 2+ and Myopia Master

Axial length became one of the most important biometric parameters in ophthalmology when cataracts started to be treated surgically. It is still one of the most important para­meters to determine the refractive power of the intraocular lens to be implanted before surgery, which reduces post­operative refractive errors.¹ In recent years, however, axial length measurement has also become increasingly important in ophthalmic optics and optometry. One reason for this is the introduction of new products in myopia management around 2020. Various metastudies predict a global increase in myopia,^2,3which particularly affects children and adolescents.³ According to projections, around half of the world‘s population will be short-sighted by 2050, 9.8 % of which will be highly myopic with an ametropia of over 5 D.³ An increased growth in axial length has been observed in myopic children compared to emmetropic children.⁴ This increase leads to a higher risk of various secondary diseases due to the associated structural changes in the eye bulb. It has been shown that axial lengths of over 26.5 mm imply a higher risk of retinal detachment, staphyloma, myopic maculopathy and glaucoma.⁵

In recent years, there has been a paradigm shift away from refraction monitoring towards axial length monitoring. Today, axial length is considered the primary parameter to monitor the progression of myopia.⁶ Various devices for measuring axial length have been available on the market since 2020 to identify children with increased axial length growth at an early stage as part of a screening procedure.

Another development that also highlighted the importance of axial length in ophthalmic optics at around the same time was the market launch of biometrically optimised ophthalmic lenses. In 2018, for example, Rodenstock GmbH (Regen) developed DNEye Pro, a procedure that incorporates biometric data from the eye when calculating lens parameters in order to improve their imaging properties. These lenses have been available under the brand name B.I.G. Exact (biometric intelligent glasses) since 2020. According to Rodenstock, the improvement in imaging properties is achieved by calculating the lens parameters on the retinal plane instead of the classic calculation on the vertex sphere. The biometric data collected is used to construct a customised eye model as the basis for calculating the interface geometry . The aim is to better map the visual areas of the progressive lens on the retina.⁷ Optiswiss AG (Basel) also launched a biometrically optimised ophthalmic lens in 2022, in which the biometric data is collected using the Myopia Master (Oculus Optikgeräte GmbH, Wetzlar).

In the case of Rodenstock lenses, the biometric para­meters are determined using the DNEye Scanner, which is distributed by Rodenstock and is identical in construction to the VX120 from Visionix Ltd. This is a multifunctional eye analyser that records objective refraction data under photopic and mesopic conditions, determines higher order aberrations for short and long range, measures the internal anterior chamber depth and central corneal thickness using the Scheimpflug method and also records pupillometry and topography in the same measurement sequence. The measurement of aberrations is carried out using a Shack-­Hartmann sensor, while topography is determined according to the Placido principle in a central area of the anterior corneal surface with a 10 mm diameter. Although the axial length and aberrations of the lens are taken into consideration when calculating the spectacle parameters, they are not measured directly but calculated analytically.⁸ The central corneal radii, corneal thickness, anterior chamber depth and objective and subjective refraction values are included in the axial length calculation. However, the underlying calculation model is not disclosed. In order to obtain the calculated axial length value, the measurement from the DNEye Scanner must be exported to an external computer software and the subjective refraction values must also be entered. The Rodenstock Consulting software and the newer CNXT platform are currently available for this purpose. A review of the literature revealed only one published study comparing the calculated axial lengths of the DNEye Scanner with the results of established biometers. In the study by Hessler et al.,⁹ the axial length of 120 eyes was determined with the DNEye Scanner and Consulting and compared with the measurements of the IOLMaster 700 (Carl Zeiss Meditec AG, Jena) and the Myopia Master. A statistically significant difference in the mean axial length difference was found between the DNEye Scanner and the Myopia Master (0.67 ± 0.46 mm, p = 0.001), as well as between the DNEye Scanner and the IOLMaster 700 (0.64 ± 0.46 mm, p = 0.001).

Due to the significant deviations in the axial lengths calculated with the Consulting software compared to the ones obtained with the established biometers and the axial-length results of the new CNXT software, which have not yet been analysed, there is a justified interest in scientifically studying the measurement results of the DNEye Scanner. The aim of this study was to examine the axial length values of the DNEye Scanner in comparison with the ones obtained by using an established biometer. We also wanted to explore whether differences in axial length arise when analysing the same measurement between the Consulting and CNXT programmes. Further goals were to compare the keratometer and objective refraction values between the DNEye Scanner and Myopia Master.

In order to achieve this, we compared the measurement results of the DNEye Scanner (axial length, refraction and central corneal radii) with those of the Myopia Master. The Myopia Master is a multifunctional eye analyser that has been on the market since 2020 and was developed for myopia screening in children. In addition to the objective refraction, the device also measures the central corneal radii and the corneal diameter using a multidot-keratometer. The axial length is measured using the dual PCI method (partial coherence interferometry). The device also contains a Scheimpflug pachymeter and myopia management software, which were, however, irrelevant for this study.

We present here a prospective, monocentric, cross-sectional study. The ethics committee of the Jena University Hospital approved this study on October 7, 2022 (Reg. No. 2021-2180-MV). The study was conducted in accordance with the conventions of the Declaration of Helsinki and the guidelines of good clinical practice (GCP).

Test subjects

62 subjects with healthy eyes aged between 20 and 71 years (47.1 ± 13.9 years) took part in this study. Before the measurements began, the study participants were informed in detail and gave their informed consent. This was followed by a medical history interview and an examination of the anterior segment of the eye using a slit lamp. Exclusion criteria were pathologies of the anterior and posterior segment of the eye, systemic diseases with a potential influence on the visual system, corrected distance visual acuity of less than 0.8 (Vcc > 0.1 logMAR), media opacity with reduction in visual acuity, fixation disorders and previous refractive surgery.

Measurements

Both eyes of the test subjects were measured three times successively on both devices within a time window of 30 minutes. The order of the measurements was randomised, and the respective manufacturer‘s recommendations were followed. Subsequently, a subjective refraction determination of both eyes was carried out; a procedure necessary for the evaluation of the axial length determination of the DNEye Scanner. The data collected included axial length, central corneal radii and objective refraction. The data from the DNEye Scanner was analysed using both the Rodenstock Consulting software (version 4.253.0.0) and the newer CNXT professional software (version 23.3.7). The “DNEye Pro” measurement procedure was selected for the measurements, in which all measurements are included except tonometry.

Statistics

After collecting all data, one eye of each subject was selected at random (randomisation using Microsoft Excel), whose data were then statistically analysed using Excel (Microsoft) and SPSS (IBM SPSS Statistics, version 28). We tested the data for normal distribution using the Kolmogorov-Smirnov test. The t-test for paired samples was used if it was confirmed that the data was indeed normally distributed; for non-normally distributed variables, the Wilcoxon signed-rank test was used. The significance level was set at α = 0.05 before the start of the study and a post-hoc Bonferroni test of the p-values was performed based on multiple testing. The mean value from the triple measurements for each parameter was calculated from the subject data collected and the median, mean
value (MV), standard deviation (SD), minimum and maximum were determined. In order to be able to compare the two measurement methods, the data was also analysed using the Bland and Altman method.¹⁰ The limits of agreement (LoA), which define the 95% confidence interval (CI) assuming normal distribution, were calculated as follows: upper LoA = MV of the differences + 1.96 × SD, lower LoA = MV of the differences − 1.96 × SD.

The refraction and keratometer data collected as polar coordinates (sphere, cylinder and axis, or central corneal refractive power and axis) were transformed into the vector components M, J₀ and J₄₅ according to Thibos et al.¹¹

In order to check the reliability of the measurement methods of both devices, the measurements of all para­meters examined were carried out three times in a row on each subject in a randomised sequence. These data were then used to calculate the intraclass correlation coefficient (ICC) and Cronbach‘s alpha (α) for both devices using SPSS (version 28). We can assume a very high reliability of the measurement method if the ICC values lie above 0.9.12 When interpreting Cronbach‘s alpha, Bland and Altman¹³ recommend to assume that a measurement system delivers reproducible results if the values are greater or equal to 0.95.

The within-subject standard deviation (Sw) was also calculated. This determines the stochastic measurement error between the individual measurements of a measurement sequence, as fluctuations around the mean value generally occur in multiple measurements. The calculation was performed using Microsoft Excel using the following formula (where s_i² corresponds to the variance of the individual measurements of a test subject and n to the number of test subjects): Sw = √ ( (∑ s_i² ) / n)

The clinical relevance of the results was assessed based on various publications.^14,15,16 For axial length, a deviation of ± 0.1 mm was classified as clinically relevant. In the case of keratometry values, these were considered clinically relevant if a deviation of ± 0.1 mm (corresponding to 0.57 D) was detected. In the case of refraction values, the criterion was a deviation of the spherical equivalent (SE = sphere + cylinder / 2) of ± 0.5 D.

In total, data from 62 test subjects (32 female and 30 male) aged between 20 and 71 years (47.1 ± 13.9 years) were collected and analysed. The refraction for the spherical equivalent (SE = Sph + Cyl / 2) lay between −12.75 and +5.00 D with a maximum cylinder value of 2.5 D. The data set was normally distributed, except for the vector component J₄₅ of the keratometry data, SE and J₄₅ of the objective refraction values and SE and J₄₅ of the subjective refraction results.

Axial length

The mean values of the axial length were 24.44 ± 1.23 mm for the DNEye Scanner analysed with Rodenstock Consulting, 24.00 ± 1.12 mm for the DNEye Scanner analysed with CNXT and 23.93 ± 1.18 mm with the Myopia Master.

DNEye Scanner Consulting vs. CNXT

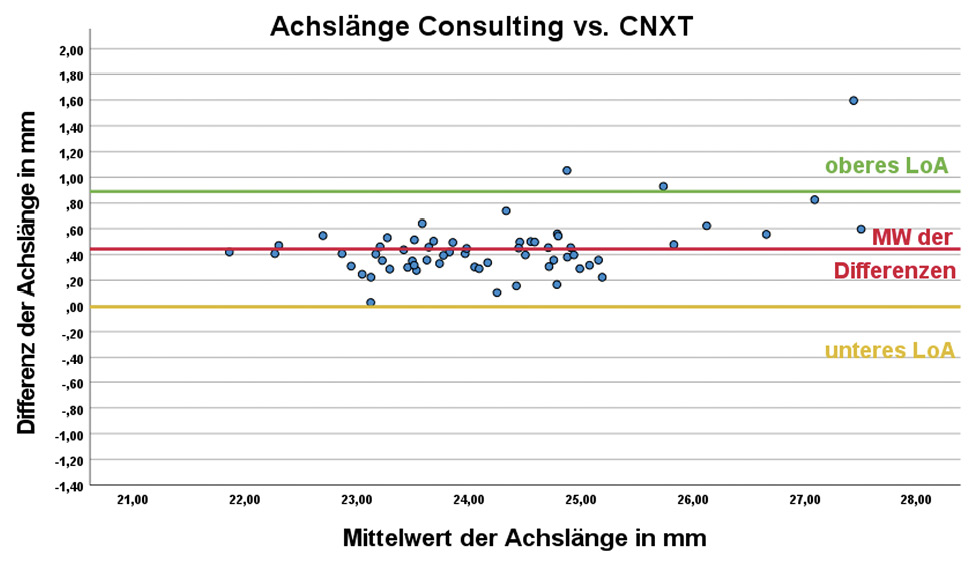
When analysing the measurements of the DNEye Scanner with the two programmes Consulting and CNXT, the mean difference in axis lengths was 0.44 ± 0.23 mm. The difference in the calculated axial lengths was statistically significant (p < 0.001). Both the difference in the mean values and their associated scatter were also clinically relevant.

DNEye Scanner Consulting vs. Myopia Master

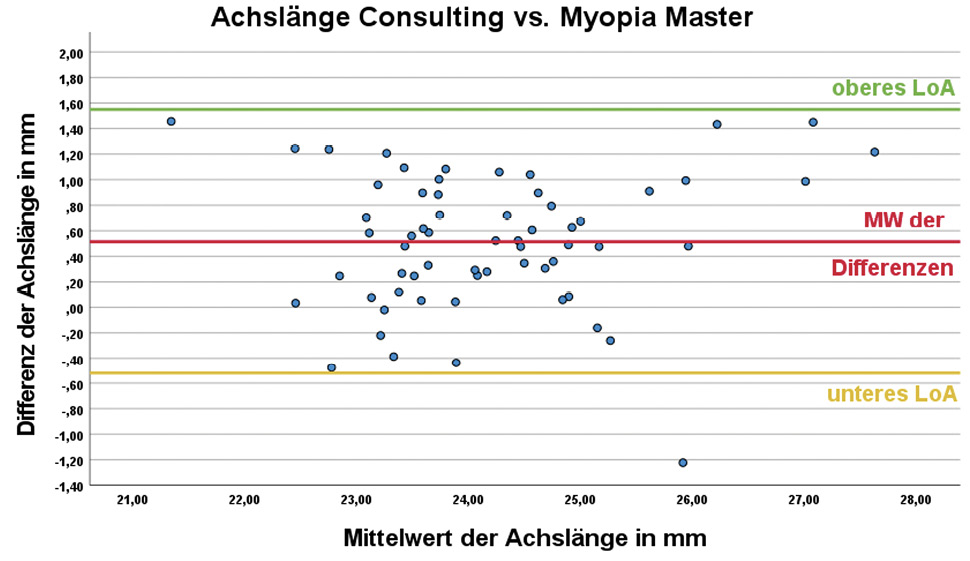
The difference between the results of the DNEye Scanner analysed with Consulting and those of the Myopia Master were also statistically significant (p < 0.001). The difference in the mean values in this case was 0.52 ± 0.53 mm and can be categorised as clinically relevant.

DNEye Scanner CNXT vs. Myopia Master

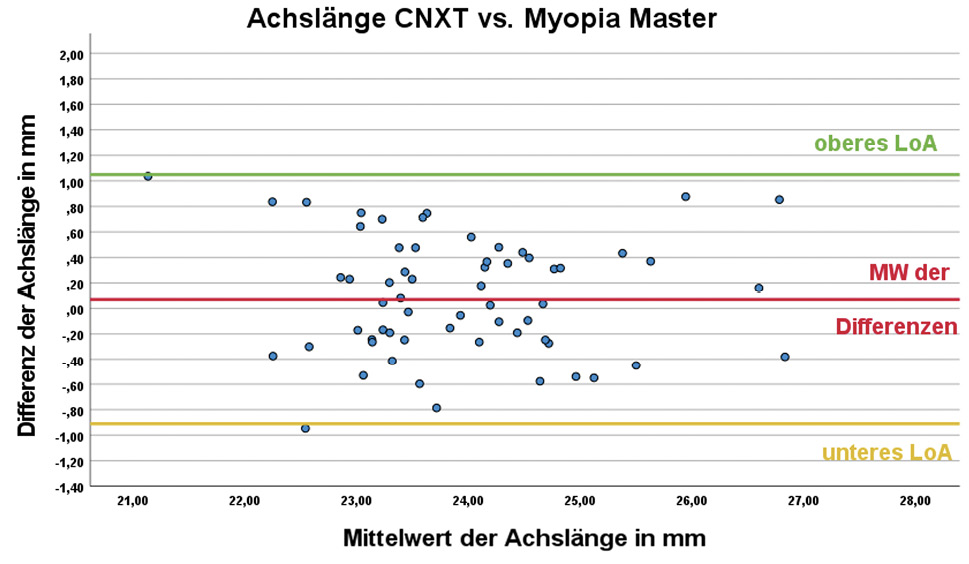
The difference in the mean values between CNXT and Myopia Master was 0.07 ± 0.50 mm. No statistically significant difference was found between the axial length values (p = 0.792). However, the difference in the axial length results between the two devices shows a scatter of 1 mm around the mean value, which corresponds to a scatter of approximately 3.33 D when converted to dioptres and should be classified as clinically relevant.¹⁷ It is well above the previously defined maximum deviation in axial length of ± 0.1 mm, which was categorised as clinically relevant, and is comparable to the dispersion of the mean value differences between Consulting and Myopia Master (0.52 ± 0.53 mm).

Table 1 summarises the results of the Bland-Altman analysis. The plots for the individual axial length differences can be found in Figure 1.

Keratometry

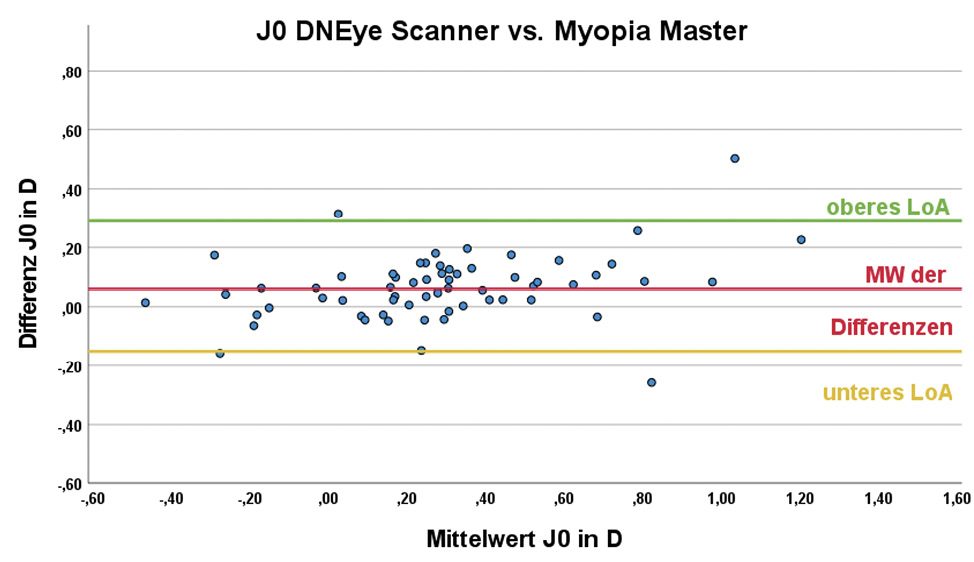
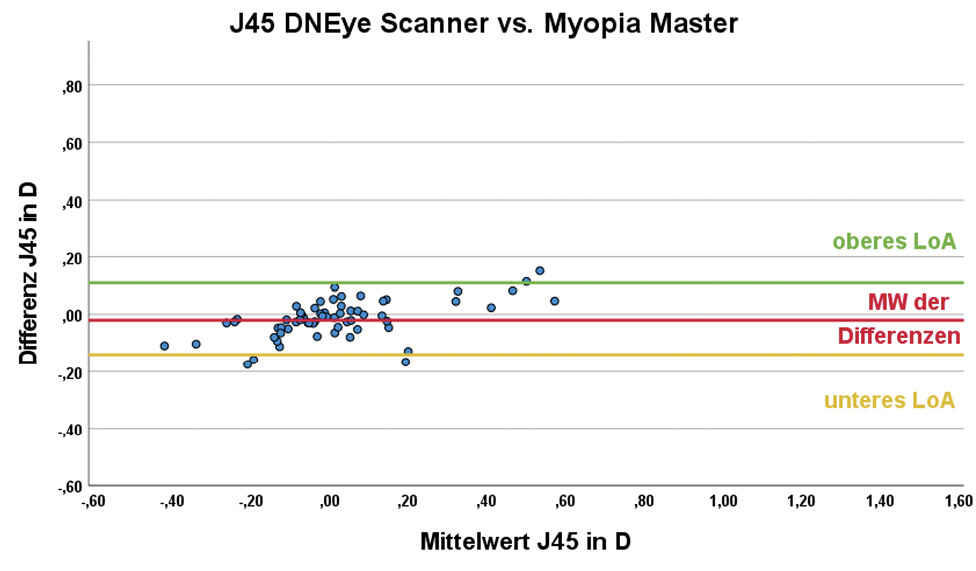
The central corneal radii outputs by the devices were transformed into the vector components Kmean , J0 and J45 prior to statistical analysis. The mean corneal refractive power Kmean was 43.35 ± 1.40 D for the DNEye Scanner 2+ and 43.34 ± 1.35 D for the Myopia Master. There is no statistically significant difference between the results of the two devices (p = 1.000). For the cylinder component J0, the mean value of the DNEye Scanner was 0.32 ± 0.35 D and that of the Myopia Master was 0.26 ± 0.32 D; being the difference statistically significant (p < 0.001). The corresponding results for the cylinder component J45 were 0.01 ± 0.22 D and 0.03 ± 0.18 D. In this case, the difference between the two devices was not statistically significant (p = 0.201). The results of the Bland-Altman analysis are summarised in Table 2; the corresponding Bland-Altman plots can be found in Figure 2.

Refraction

The objective refraction values of sphere, cylinder and axis output by the devices were transformed into the vector components spherical equivalent (SE), J0 and J45 prior to statistical analysis. The mean value of the SE was −1.11 ± 2.84 D for the DNEye Scanner and −1.01 ± 2.79 D for the Myopia Master; the difference was statistically significant and had a value of −0.10 ± 0.22 D (p = 0.003). For the cylinder component J0 , the result of the DNEye Scanner was 0.07 ± 0.43 D and that of the Myopia Master −0.01 ± 0.40 D. The difference between the devices is statistically significant (p < 0.001). The corresponding results for the cylinder component J45 were −0.02 ± 0.27 D and 0.03 ± 0.24 D and also show a statistically significant difference (p = 0.003). Despite the statistically significant difference in SE, the difference is in a clinically acceptable range of less than ± 0.5 D. The results of the Bland-Altman analysis are summarised in Table 3.

Reproducibility of the results

In order to check the repeatability of the two devices, the ICC, the within-subject standard deviation and Cronbach‘s alpha were calculated for all measured parameters.

Axial length

Table 4 summarises the findings for the repeatability of the axial length results. Both the Rodenstock evaluation programmes in combination with the DNEye Scanner as well as the Myopia Master showed very good reproducibility with an ICC of ≥ 0.996 and values for Cronbach‘s alpha ≥ 0.997. The within-subject standard deviation was 0.02 mm for the Myopia Master and 0.08 mm for DNEye Scanner when analysed with CNXT. When analysed with Consulting, this parameter was 0.10 mm and can therefore be classified as clinically relevant. This means that the fluctuations of the repeated measurements around the mean value were smaller with the Myopia Master than with the DNEye Scanner, which therefore delivered more precise values.

Keratometry

For keratometry, the test for repeatability also showed very good reproducibility of the results for both devices. As can be seen in Table 5, the Myopia Master achieved slightly higher values for the ICC and Cronbach‘s alpha (except for J0) and therefore an even higher reliability than the DNEye Scanner. The within-subject standard deviation is somewhat lower for the Myopia Master than for the DNEye Scanner, which means a smaller fluctuation of the measured values around the mean for the measurements repeated three times and indicates a smaller measurement error between these.

Refraction

The repeatability of the objective refraction values showed an ICC > 0.950 for both devices and all three vector components and therefore a very good reproducibility of the results. Cronbach‘s alpha was 0.999 for both devices for the SE, whereas the cylinder components yielded values between 0.978 and 0.990 (see Table 6). The within-subject standard deviation for the DNEye Scanner was 0.11 D for the SE, 0.06 D for J₀ and 0.03 D for J₄₅ . For the Myopia Master, it was 0.12 D for the SE, 0.07 D for J₀ and 0.05 D for J₄₅ .

In this study, we compared the results for axial length, keratometry (Kmean , J₀ , J₄₅) and refraction (SE, J₀ , J45) for the devices DNEye Scanner 2+ and the Myopia Master and the reproducibility of the measurement results of both was checked.

Axial length

The axial lengths determined with the DNEye Scanner in combination with the Consulting software show significant differences compared to the evaluation with the newer CNXT software as well as to the results of the Myopia Master.
Although the mean difference between the axial lengths of the DNEye Scanner analysed with CNXT and the Myopia Master is not statistically significant (p = 0.792), the scatter of the results (1 mm) around the mean value (0.07 ± 0.50 mm) is clinically relevant. There are already comparative studies on the Myopia Master in which the axial length was compared to that obtained with the IOLMaster as the established gold standard. Ye. et al. (2022)18 published a mean difference of 0.01 mm (with a 95% CI of LoA of [−0.09; 0.12], p = 0.908) between IOLMaster 500 and Myopia Master. Further studies comparing the Myopia Master with the IOLMaster 700 showed comparable mean axial length differences of −0.004 ± 0.047 mm (p = 0.34) 18 and −0.02 ± 0.02 mm (p = 0.89).⁹

At present, only the study by Hessler et al. (2023)9 compares the axial length determined with the DNEye Scanner ² and the Consulting software with the results of the Myopia Master and the IOLMaster 500. The study provides comparable results to this study (0.52 ± 0.53 mm, p < 0.001) in a sample of 120 eyes with a mean difference between Myopia Master and DNEye Scanner of 0.67 ± 0.46 mm (p = 0.001). The mean difference in axial length between IOLMaster 700 and DNEye Scanner is 0.64 ± 0.46 mm (p = 0.001). The determination of the axial length of the DNEye Scanner with the Consulting software provided clinically inadequate results in both studies.

The newer CNXT software appears to use a different or modified formula for calculating the axial length, as the comparison of the evaluation of the same measurements on the DNEye Scanner showed a difference in the mean values between Consulting and CNXT of 0.44 ± 0.23 mm, which was statistically significant (p < 0.001). In the Bland-Altman plot (Figure 1), the left graph (Consulting vs. CNXT) shows a discrepancy between the results of the two programmes that increases with increasing axial length starting at an axial length of 26 mm.

Although the mean axial length values determined with CNXT do not differ significantly from those of the Myopia Master (p = 0.792), the considerable scatter of 1 mm of the 95% CI is clinically relevant and comparable to the scatter with the Consulting software. Due to the large discrepancy between the axial length results of CNXT compared to those of the Myopia Master as a reference, the use of the axial lengths predicted with CNXT in the context of biometric measurements, such as in myopia management, is not recommended. In practice, it is therefore advisable not to compare axial length results from the DNEye Scanner with results from other devices, nor to compare the axial length results analysed with the two different programmes Consulting and CNXT.

Keratometry

No statistically significant difference was found for the mean corneal refractive power Kmean between the measurements of the two devices. The cylinder component, J₀ , differs significantly between the two devices, but in the clinically insignificant range of 0.07 ± 0.11 D. The vector component, J₄₅ , does not differ significantly between the two devices.

In the study by Hessler et al. (2023),9 there was also no statistically significant difference between the Kmean values of the two devices. The study by Pedersen et al. (2023)¹⁹ compared the values of Kmean of the Myopia Master and the IOLMaster 700. The mean difference here was 0.035 ± 0.028 mm (p < 0.001) and resulted in significantly flatter mean corneal radii for the Myopia Master. In a further study by Zhang et al. (2019),20 the VX120, which is identical in construction to the DNEye Scanner, was compared with the Pentacam (Oculus Optikgeräte GmbH). The mean values for Kmean were as follows: 43.42 ± 1.63 D for the VX120 and 43.20 ± 1.69 D for the Pentacam. The difference in said study was statistically significant (p < 0.001).

Since in this study neither the mean differences between the two devices nor the corresponding scatter exceed the previously defined limit value of ± 0.57 D (this corresponds to a radius difference of ± 0.1 mm), both devices are suitable for determining the central corneal radii in optometric practice.

Refraction

At −1.11 ± 2.84 D, the spherical equivalent determined with the DNEye Scanner produced slightly more negative values than the Myopia Master (−1.01 ± 2.79 D). The difference is statistically significant (p = 0.003), but is within a clinically acceptable range due to the difference between the values of −0.10 ± 0.22 D. Compared to the subjective refraction determination, the objective values for the SE of the DNEye Scanner with a mean difference of 0.09 ± 0.20 D are closer to the subjective result than those of the Myopia Master with 0.19 ± 0.22 D.

The cylinder components J₀ and J₄₅ showed significant deviations between the two devices. However, as the mean difference is less than 0.08 D, the divergence is not clinically relevant. If we compare the vector components J₀ and J₄₅ for objective and subjective refraction determination, we find a statistically significant difference in the cylinder component J0 for the DNEye Scanner and J₄₅ for the Myopia Master. However, the difference in the mean values is less than 0.05 D, meaning there is no clinical relevance here either.

In an earlier study, Gordon-Shaag et al.21 published the following mean differences for the vector components of the subjective refraction when comparing the VX120, which is identical in construction to the DNEye Scanner: SE 0.14 ± 0.47 D (p = 0.82), J0 0.10 ± 0.18 D (p < 0.01) and J45 -0.05 ± 0.17 D and thus presented a slightly larger deviation than in this study.

In their study, Ye et al.¹⁸ determined a mean SE value of −1.35 ± 2.12 D for the Myopia Master and −0.93 ± 2.13 D for the ARK-1 autorefractokeratometer (Nidek Co, Ltd.). Comparatively, the subjective refraction determination resulted in −0.86 ± 2.11 D and was in better agreement with the values of the ARK-1.

As both the mean differences of the spherical equivalent and the corresponding scatter between the DNEye Scanner and Myopia Master are below the previously defined value of ± 0.50 D, both devices are suitable for determining objective refraction values in optometric practice. Compared with the subjective refraction, the mean difference and scatter are also less than ± 0.50 D. They are also comparable with the results of other established autorefractometers.

Repeatability

Axial length

Both devices exhibit very good reproducibility of the
axial length measurements. Analysed with Consulting, the DNEye Scanner delivers an ICC of 0.996, while the value for CNXT is 0.997. An excellent repeatability with an ICC of 1.0 was determined for the Myopia Master. Cronbach‘s alpha is 0.997 for both analyses with the DNEye Scanner and 1.0 for the Myopia Master. The results are comparable with those of a study by Hashemi et al. (2022),22 who investigated the reproducibility of the results for the Galilei G6 optical biometer (Ziemer Group) and compared them to the results of the IOLMaster 500. The ICC values in that study were 0.995 for AL < 22 mm, 0.999 for AL 22 – 24 mm and 0.999 for AL > 24 mm.

The within-subject standard deviation is 0.02 mm for the Myopia Master, 0.10 mm for the DNEye Scanner with Consulting and 0.08 mm with CNXT. This means that the deviations within the three repeated measurements are smallest for the Myopia Master.

Keratometry

The DNEye Scanner showed an ICC ≥ 0.95 for all three vector components of keratometry and therefore very good reproducibility of the results. Cronbach‘s alpha takes values ≥ 0.99 for the three vector components.

For the Myopia Master, the ICC values were ≥ 0.971. The mean corneal refractive power Kmean reached a slightly higher value of 0.997 for both the ICC and alpha than the DNEye Scanner with 0.995 in each case.

These results are consistent with other studies on the measurement accuracy of the two devices. Gordon-Shaag et al.²¹ published ICC values for the VX120 of 0.984 for the flat corneal meridian and 0.988 for the steep corneal meridian. Ye et al.¹⁸ investigated the reproducibility of the Myopia Master and published an ICC of 0.977 for Kmean .

Refraction

The repeatability of the objective refraction values for both devices is in a very satisfactory range of ICC > 0.97 for all three vector components SE, J₀ and J₄₅ . The values for Cronbach‘s alpha are also above a value of 0.97 for all vector components.

The ICC values determined in this study are comparable with the results of Gordon-Shaag et al.,21 who determined ICC values of SE = 0.995, J0 = 0.972, J45 = 0.970 for the VX 120.

Both devices are suitable for recording keratometry measurement values in optometric practice thanks to their measurement accuracy. The same applies to the measurement of objective refraction values. Here too, the obtained values are comparable with those of other established auto­refractometers.

Despite a non-significant difference in the mean values, axial lengths determined with CNXT should not be compared with results from Myopia Master due to the considerable and clinically relevant scatter and should not be used in the context of myopia management. This also applies to axial lengths determined with the Consulting software. The DNEye Scanner and the Myopia Master are not interchangeable in terms of axial length determination.

Both devices have a high reproducibility of the measurement results for all tested parameters (axial length, vector components of keratometry and objective refraction).

Conflict of interest

Philipp Hessler and Stephan Degle are consultants at the Oculus Academy. Stephan Degle is also consultant at the Rodenstock Akademie.